Borromean Glow Stick Bracelets

As a (slightly belated) Halloween post, here's a cool thing you can do with glow stick bracelets (or really anything, I just happen to have a lot of these now) -

Check it out! This arrangement is known as the Borromean rings and is particularly interesting because no two rings are actually linked to each other, yet all three together are linked. They can be worn like so in a nice, mathematically interesting bracelet.

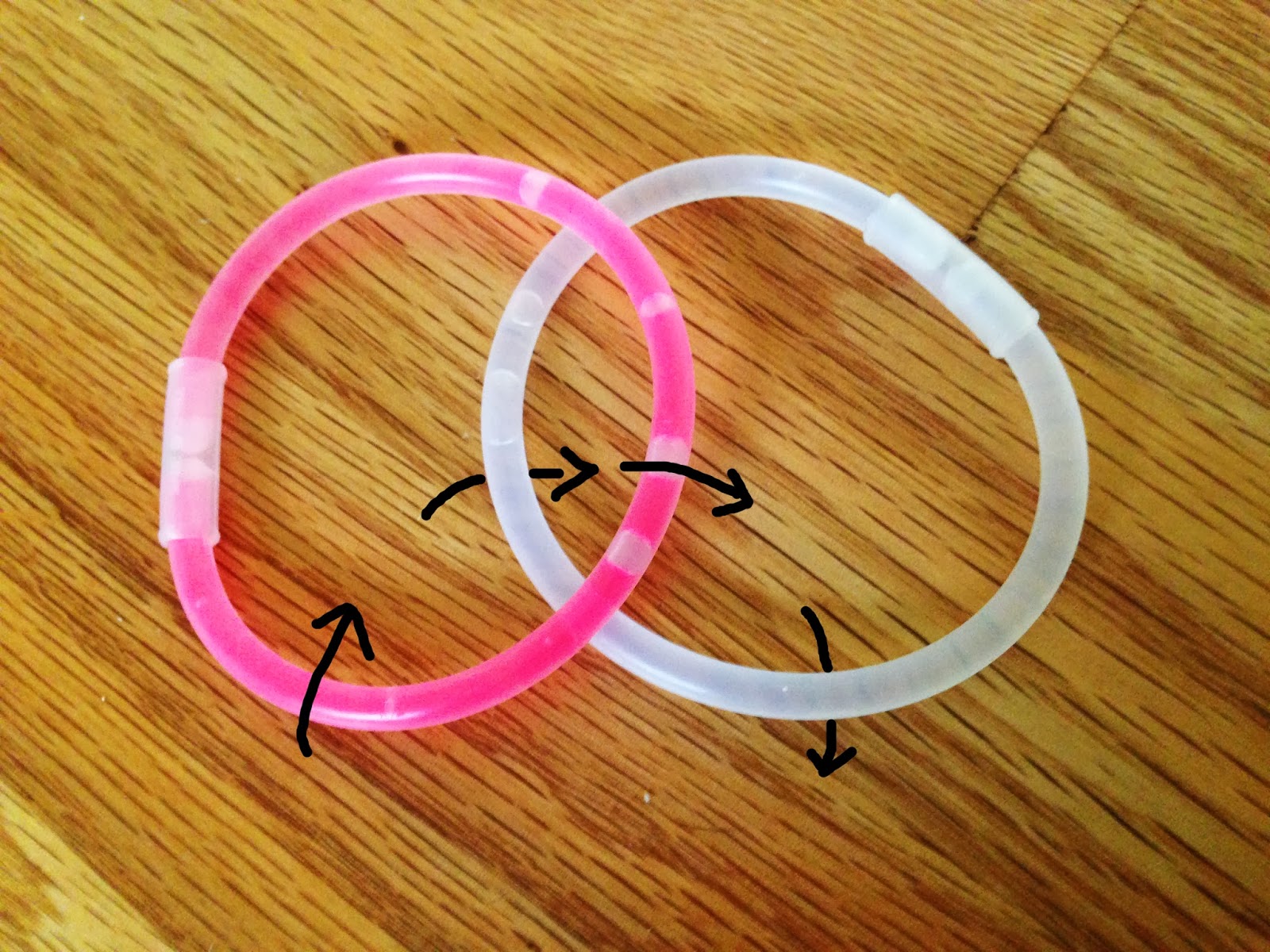
Putting these rings together is fantastically simple. First take two bracelets (or other circular objects of your choice) and overlap one on the other like so.

Then, take the third bracelet and weave it through the other two, alternating over and under as you come around. 

Then simply connect up the two ends of the third bracelet and voila!

If you look closely, you'll find some other neat properties. For example, if you were to cut the Borromean rings, you would get one iteration of the standard three-strand braid, suggesting an alternate method of construction. Just as if you were to remove one strand of a braid and cause it to fall apart, you can try and take out one ring and see that the other two are no longer linked. 

Also notice how if you look at any one ring, it is wholly inside of, and wholly outside of, the other two rings.

Alternatively, you could dip your rings into some bubble solution. The result is one of a class of objects called Seifert surfaces, any surface defined by a knot or link. Here is a beautiful sculpture of this surface (image source) 

And just think, all of this from three simple rings... 

 

"I'm just playing. That's what math is - wondering, playing, amusing yourself with your imagination." - Paul Lockhart