Twist - Exploring the Cube and its Faces

A recent sculptural undertaking of mine - although it may not seem like it at first, this tangled mess is actually based upon the cube. It's made of 24 empty pen barrels, tied together with rubber bands. There are six square faces, two of each color (the faces of the same color are parallel). Essentially, it is what I think would happen if you took a cube, pushed its square sides inward, and gave them a twist. Below is a single square face -

Then, each of the three pairs of faces are arranged with a π/4 twist, which looks like this when viewed face on -

This arrangement makes eight convenient slots where the other four faces can interlock, as shown here. The darker/opaque green and purple dots indicate how the green and purple squares fit into the blue ones. Imagine that the set of green faces and the set of purple faces both also have a half twist to them. Also, the lighter green and purple dots on the intersection of the green and purple pieces drawn indicate the corners of the faces (the green dots on the purple pieces means the green overlaps there and two green sides come together at that point as a corner, and vice versa for the purple dots on the green pieces). You can actually see this a bit in the first picture of the actual sculpture, where the green and blue faces are held in place by the purple, and how the corners of the blue and green meet up and overlap.

If you turned the piece around, you would see this arrangement three times, once with each color serving as the outer "framework" as the blue is shown here. This was actually quite challenging to assemble, as it became a feat of intense coordination to hold everything together before it was all tied in place, but finished up, it pretty much holds itself together now. I made each of the squares smaller to tighten up the structure and I like how the ends sticking out at each corner give the whole thing a messier look - a curious juxtaposition of order and chaos.

Borromean Glow Stick Bracelets

As a (slightly belated) Halloween post, here's a cool thing you can do with glow stick bracelets (or really anything, I just happen to have a lot of these now) -

Check it out! This arrangement is known as the Borromean rings and is particularly interesting because no two rings are actually linked to each other, yet all three together are linked. They can be worn like so in a nice, mathematically interesting bracelet.

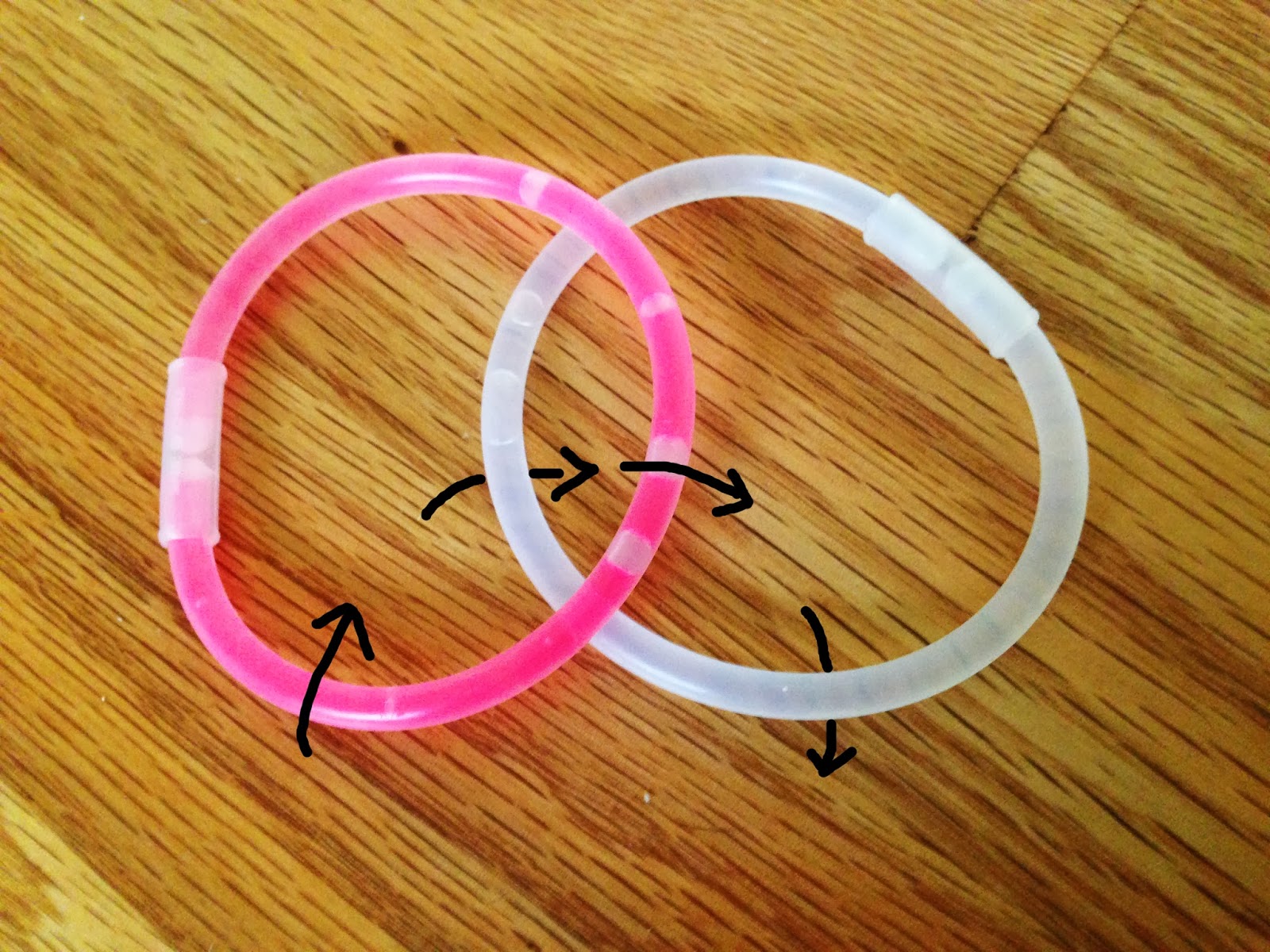
Putting these rings together is fantastically simple. First take two bracelets (or other circular objects of your choice) and overlap one on the other like so.

Then, take the third bracelet and weave it through the other two, alternating over and under as you come around. 

Then simply connect up the two ends of the third bracelet and voila!

If you look closely, you'll find some other neat properties. For example, if you were to cut the Borromean rings, you would get one iteration of the standard three-strand braid, suggesting an alternate method of construction. Just as if you were to remove one strand of a braid and cause it to fall apart, you can try and take out one ring and see that the other two are no longer linked. 

Also notice how if you look at any one ring, it is wholly inside of, and wholly outside of, the other two rings.

Alternatively, you could dip your rings into some bubble solution. The result is one of a class of objects called Seifert surfaces, any surface defined by a knot or link. Here is a beautiful sculpture of this surface (image source) 

And just think, all of this from three simple rings... 

 

"I'm just playing. That's what math is - wondering, playing, amusing yourself with your imagination." - Paul Lockhart